[mathjax]
Let’s take a look at this A maths question on Equations and Inequalities. A handful of students will be confused with the keywords or how to effectively determine the correct sign of the discriminant.
The example below is very similar to St Margaret 2016 A Math Prelim Paper 1 Q2.
Original Question: Find the range of values of [latex]p[/latex] for which [latex](p+2)x^2 – 12x + 2(p-1)[/latex] is always negative.
Modified Example: Find the range of values of [latex]p[/latex] for which [latex](p+2)x^2 – 12x > -2(p-1)[/latex] for all real values of [latex]x[/latex].
Misconception:
Students will mistaken REAL as the keyword and likely think that [latex]b^2-4ac \geq 0[/latex].
The emphasis is that the word REAL is crucial ONLY if coupled with a friend called ROOTS. We need to see the Keyword – REAL ROOTS for [latex]b^2-4ac \geq 0[/latex] to hold true.
Step 1:
Ensure that the right hand side of the inequality is zero.
[latex](p+2)x^2 -12x + 2(p-1) > 0[/latex]
Step 2:
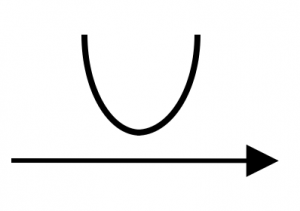
Having done so, recognise the keyword as “[latex]>0[/latex]”. This implies that the graph will be above the axis and the shape of this quadratic expression will be a Happy Face. Since the U-shape is not intersecting with the axis, the solutions will be imaginary and [latex]b^2-4ac < 0[/latex].
Keyword [latex]>0[/latex] implies
[latex]\Rightarrow[/latex] Happy Face is ABOVE axis
[latex]\Rightarrow[/latex] No Real Solutions
[latex]\Rightarrow[/latex] [latex]b^2-4ac < 0[/latex]
Step 3:
Observe [latex]a=p+2, b=-12[/latex] and [latex]c=2p-2[/latex]
For imaginary solutions, we have :
[latex]b^2-4ac<0[/latex]
[latex](-12)^2-4(p+2)(2p-2)<0[/latex]
[latex]144-4(2p^2-2p+4p-4)<0[/latex]
[latex]-8p^2-8p+160<0[/latex]
[latex]-p^2-p+20<0[/latex]
[latex](-p+4)(p+5)<0[/latex]
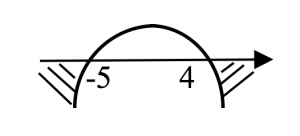
[latex]p<-5[/latex] or [latex] p>4[/latex]…..(1)
At this stage, most students are contented with getting the solutions and will think that the question is completed.
Well, we are almost there. This is a typical example of having a variable coefficient ([latex]p+2[/latex]) in front of [latex]x^2[/latex].
Extra Step is mandatory
Remember we mentioned earlier that the graph is a Happy Face, thus we expect the coefficient of [latex]x^2[/latex] to be positive and we have
[latex]p+2>0[/latex]
[latex]p>-2[/latex]….. (2)
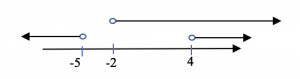
Visualise the overlapping region of answers on a number line from (1) and (2),
Therefore [latex]p>4[/latex] (final solution)